Approximate Methods for Multiple Degree of Freedom Systems: Rayleigh’s Quotient
For a conservative MDOF system, the total mechanical energy ![]() is a constant so that
is a constant so that
![]()
or using the representations in (9.1) and (9.2)
(9.3) ![]()
Now consider the system vibrating in one of its normal modes at a natural frequency ![]() . The amplitudes of the coordinates are given by
. The amplitudes of the coordinates are given by
![]()
where ![]() is the corresponding mode shape and
is the corresponding mode shape and ![]() is a constant. The derivatives of the coordinates will therefore be
is a constant. The derivatives of the coordinates will therefore be
![]()
The coordinates will have maximum values given by
(9.4) ![]()
which all occur simultaneously (when ![]() ). At the same instant,
). At the same instant, ![]() so the derivatives of the coordinates will all be zero (
so the derivatives of the coordinates will all be zero (![]() ).
).
Similarly the coordinate derivatives will have maximum values given by
(9.5) ![]()
when ![]() . At this instant,
. At this instant, ![]() so the coordinates themselves are all zero (i.e. the system is passing through its equilibrium configuration).
so the coordinates themselves are all zero (i.e. the system is passing through its equilibrium configuration).
In summary
![]()
Now, consider equation (9.3) at the instant when ![]() . We see that
. We see that
![Rendered by QuickLaTeX.com \begin{align*}\frac{1}{2} \bigl\{\!\dot{q}\!\bigr\}\!\rule[4mm]{0pt}{0pt}^{T} \!\!\bigl[m\bigr] \! \bigl\{\!\dot{q}\!\bigr\} +\frac{1}{2} \bigl\{\!q\!\bigr\}\!\rule[4mm]{0pt}{0pt}^{T} \!\!_{\text{MAX}}\!\bigl[k\bigr] \! \bigl\{\!q\!\bigr\}_{\text{MAX}} &= E \nonumber \\\frac{1}{2} \Bigl(A \!\bigl\{\!\Phi\!\bigr\}\!\rule[4mm]{0pt}{0pt}^{T} \!\Bigr) \!\!\bigl[k\bigr] \! \Bigl(\! A \bigl\{\!\Phi\!\bigr\} \!\Bigr) &= E\end{align*}](https://engcourses-uofa.ca/wp-content/ql-cache/quicklatex.com-0c1883c57e414c3b78d588ca92b1e5b1_l3.png)
or
(9.6) ![]()
Similarly, when ![]() we find
we find
![Rendered by QuickLaTeX.com \begin{align*}\frac{1}{2} \bigl\{\!\dot{q}\!\bigr\}_{\text{MAX}}\!\bigl[m\bigr]\! \bigl\{\!\dot{q}\!\bigr\}_{\text{MAX}} +\frac{1}{2} \bigl\{\!q\!\bigr\}\!\rule[4mm]{0pt}{0pt}^{T} \!\!\bigl[k\bigr] \! \bigl\{\!q\!\bigr\} &= E \\\frac{1}{2} \Bigl( \! A p \bigl\{\!\Phi\!\bigr\}\!\rule[4mm]{0pt}{0pt}^{T} \!\Bigr) \!\!\bigl[m\bigr] \! \Bigl(\! A p \bigl\{\!\Phi\!\bigr\} \!\Bigr) &= E\end{align*}](https://engcourses-uofa.ca/wp-content/ql-cache/quicklatex.com-00730dcc6e2ea07779a42f7e696b4044_l3.png)
or
(9.7) ![]()
Since the total energy ![]() is a constant for this conservative system, comparing equations (9.6) and (9.7 ) leads to the conclusion that
is a constant for this conservative system, comparing equations (9.6) and (9.7 ) leads to the conclusion that
![]()
or
(9.8) ![Rendered by QuickLaTeX.com \begin{equation*} \boxed{p^2 = \frac{\hspace{-1cm}\bigl\{\!\Phi\!\bigr\}\!\rule[4mm]{0pt}{0pt}^{T} \!\!\bigl[k\bigr] \! \bigl\{\!\Phi\!\bigr\} \rule{-3mm}{0pt}}{\bigl\{\!\Phi\!\bigr\}\!\rule[4mm]{0pt}{0pt}^{T} \!\!\bigl[m\bigr] \! \bigl\{\!\Phi\!\bigr\} \rule{5mm}{0pt}}}\end{equation*}](https://engcourses-uofa.ca/wp-content/ql-cache/quicklatex.com-bc5d3fda54d04bb096f88151aca21024_l3.png)
Equation (9.8) is known as Rayleigh’s Quotient. This result tells us that if a mode shape corresponding to a particular natural frequency is used in the ratio in (9.8), then the result will be the associated natural frequency squared.
By itself this is not a particularly useful result since typically, knowing ![]() and
and ![]() for a particular system, we must find
for a particular system, we must find ![]() as part of the process of determining the mode shapes in the first place. However, equation (9.8) can be useful for obtaining approximate natural frequencies for MDOF systems. In essence, what we do is estimate a mode shape
as part of the process of determining the mode shapes in the first place. However, equation (9.8) can be useful for obtaining approximate natural frequencies for MDOF systems. In essence, what we do is estimate a mode shape ![]() for the system and Rayleigh’s Quotient returns an associated estimate of the natural frequency. Obviously, the choice of
for the system and Rayleigh’s Quotient returns an associated estimate of the natural frequency. Obviously, the choice of ![]() will influence the value of the returned natural frequency, however it can be shown that if
will influence the value of the returned natural frequency, however it can be shown that if ![]() are the
are the ![]() natural frequencies on an
natural frequencies on an ![]() degree of freedom system, then for any assumed mode shape
degree of freedom system, then for any assumed mode shape ![]() the frequency
the frequency ![]() returned by Rayleigh’s Quotient satisfies
returned by Rayleigh’s Quotient satisfies
![]()
i.e. the frequency returned by Rayleigh’s Quotient will always be higher than the lowest natural frequency and lower than the highest natural frequency in the system. Typically we are more concerned with the lowest (fundamental) frequency in a system, for which Rayleigh’s Quotient establishes an upper bound. (This frequency also provides a lower bound on the highest natural frequency of a system but this is usually of less interest.)
Remarkably good estimates of the fundamental natural frequency can be obtained even if the assumed mode shape ![]() (also called a trial vector) is not equal to the actual first mode shape.
(also called a trial vector) is not equal to the actual first mode shape.
EXAMPLE
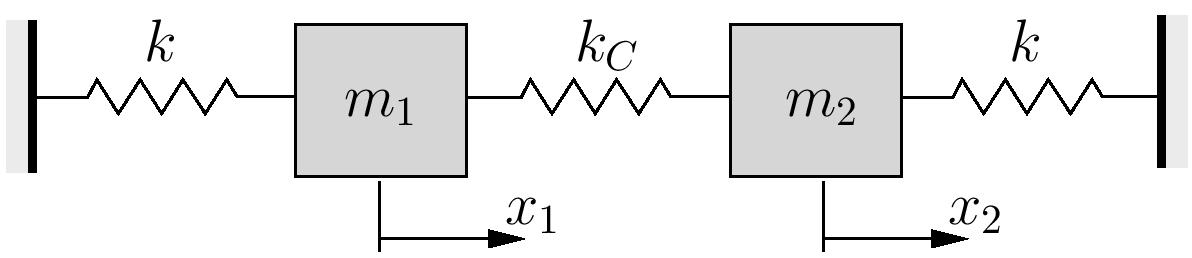
(a) For the system shown above, estimate the fundamental natural frequency using Rayleigh’s Quotient. Assume ![]() and use trial vectors of
and use trial vectors of
![]()
and comment on the results. It was previously determined that
![]()
![]()
(b) Repeat the calculations in part (a) assuming ![]() ,
, ![]() , and
, and ![]() .
.
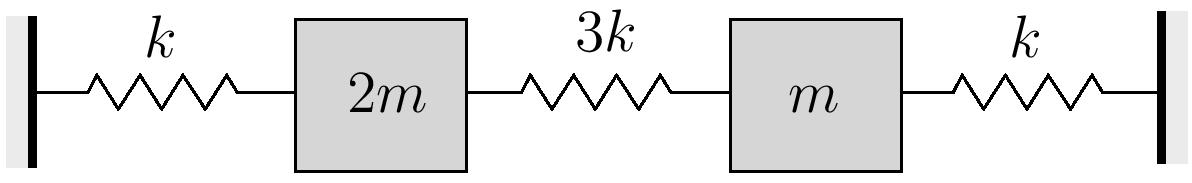
Note that the exact solution in this case is
![]()
![]()
EXAMPLE
Use Rayleigh’s method to estimate the fundamental natural frequency for a light simply supported beam supporting three equally spaced identical masses.
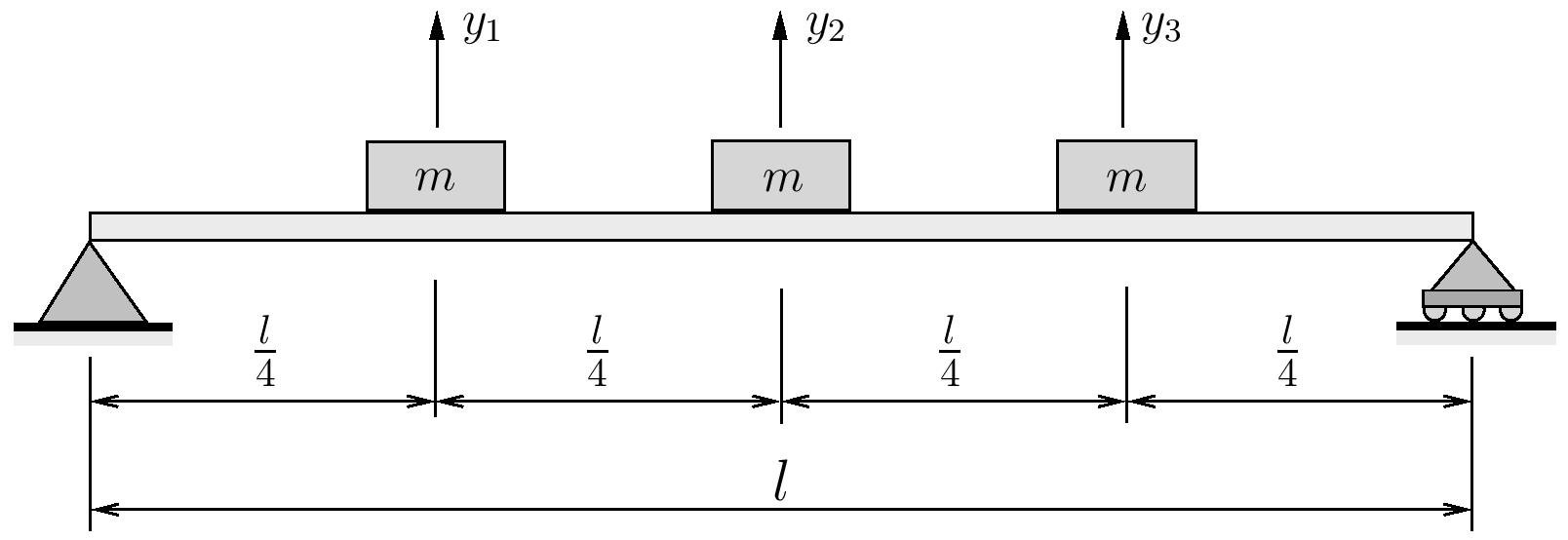
Assume trial vectors of
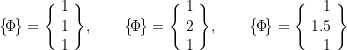
Note that for this problem
![Rendered by QuickLaTeX.com \begin{align*}\bigl[ k \bigr] &=\frac{192 EI}{7 l^3}\Biggl[\begin{array}{rrr}23 & -22 & 9\\-22 & 32 & -22\\9 & -22 & 23\\\end{array}\vspace{4mm}\Biggr] \\\bigl[ m \bigr] &=m\Biggl[\begin{array}{ccc}1 & 0 & 0\\0 & 1 & 0\\0 & 0 & 1\\\end{array}\Biggr] \\\end{align*}](https://engcourses-uofa.ca/wp-content/ql-cache/quicklatex.com-e0b3cf362fb65699d432aedc2502b00d_l3.png)

Thank you. I am 82. At seven years of age I was labeled a total retard, unteachable and unlearnable by the Catholic Church. A nun, Sister Angela Cool did an experiment and I was allowed into regular class. Throughout life I have been denounced as an idiot savant. So what? We learn differently; with the main obstacle being being libeled as above; then removed from any and all stimuli including social interaction. Leave us alone. Let us learn. And we can really contribute to society and government in our own ways. That includes keeping the self-righteous, self-promoting, shady law enforcement and other such secretive organizations off our backs. My life was destroyed by two such FBI creatures, snake-headed skunks, that claimed I did not have the imagination to develop weapons and other things for the Navy. They robbed me of credit, merit, monetary awards, and my life to make themselves safe careers. Please see that it does not happen to other people like me. So I have a hobby of learniong through self research. And my country is robbed of the benefits.