Problems: Chapter 7
1- Find the shear forces and bending moments at the sections shown. For the bending moment at S3 consider sections right before and after S3.
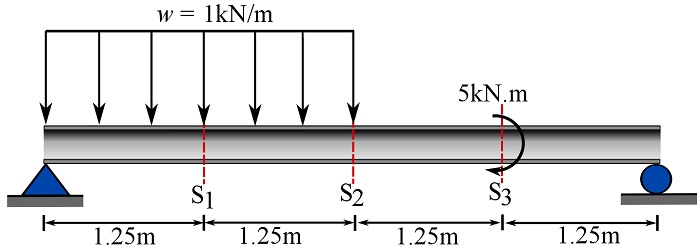
Answer
Section 1 at ![]() :
: ![]() .
.
Section 2 at ![]() :
: ![]() .
.
Section 3 at ![]() :
: ![]() .
.
2- Find the shear forces and bending moments at the sections shown.
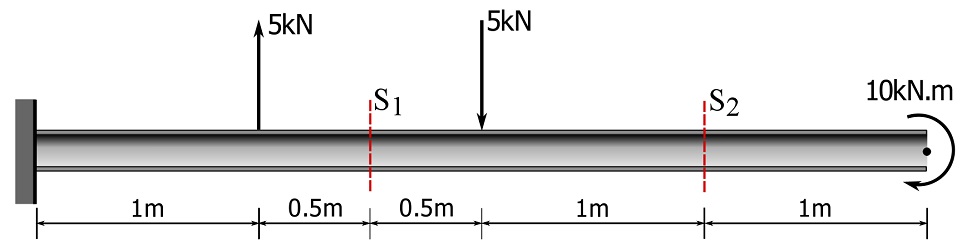
Answer
Section 1 at ![]() :
: ![]() .
.
Section 2 at ![]() :
: ![]() .
.
3- Find the shear forces and bending moments at the sections shown.
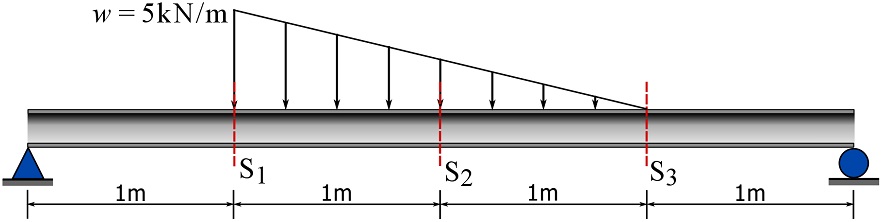
Answer
Section 1 at ![]() :
: ![]() .
.
Section 2 at ![]() :
: ![]() .
.
Section 3 at ![]() :
: ![]() .
.
4- Find the shear forces and bending moments at the sections shown.
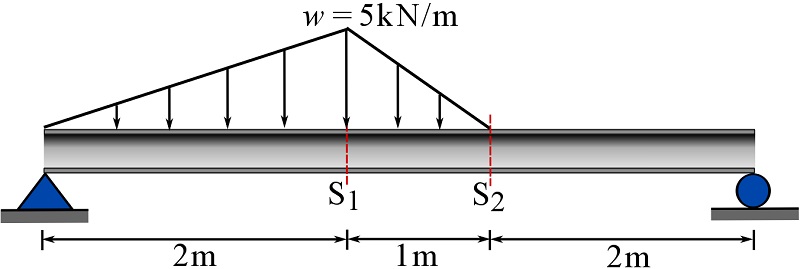
Answer
Section 1 at ![]() :
: ![]() .
.
Section 2 at ![]() :
: ![]() .
.
5- Determines the functions ![]() and
and ![]() .
.
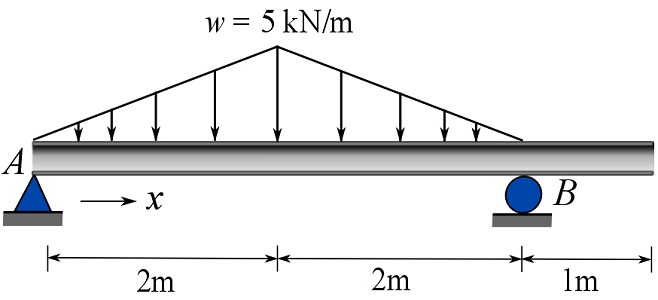
Answer
![Rendered by QuickLaTeX.com \[V(x)=\begin{cases}5 -\frac{5x^2}{4}\text{ kN} \quad \text{for } 0< x \le 2\\\frac{5x^2}{4}-5\text{ kN} \quad \text{for } 2\le x < 4\\0.0\text{ kN} \quad \text{for } 4< x \le 5\end{cases}\]](https://engcourses-uofa.ca/wp-content/ql-cache/quicklatex.com-4a6fcd674e962cb15105b7f246c01b2d_l3.png)
![Rendered by QuickLaTeX.com \[M(x)=\begin{cases}5x - \frac{5x^3}{12}\text{ kN.m} \quad \text{for } 0\le x \le 2\\\frac{5x^3}{12}-5x + \frac{20}{3}\text{ kN.m} \quad \text{for } 2\le x \le 4\\0.0\text{ kN.m} \quad \text{for } 4\le x \le 5\end{cases}\]](https://engcourses-uofa.ca/wp-content/ql-cache/quicklatex.com-5fc36504771d08cd721f8154d73ce5cb_l3.png)
6- Determines the functions ![]() and
and ![]() . Consider the support at
. Consider the support at ![]() as a roller in a slot if the support reaction becomes a pulling force there.
as a roller in a slot if the support reaction becomes a pulling force there.
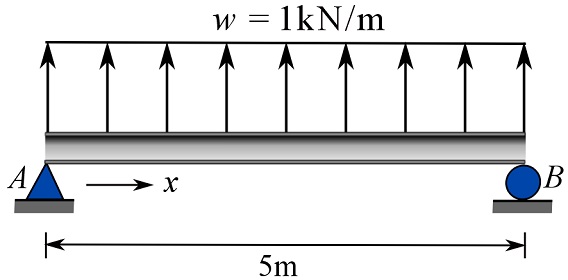
Answer
![Rendered by QuickLaTeX.com \[\begin{split}V(x)&=x -2.5\text{ kN} \quad \text{for } 0< x < 5\\M(x)&=\frac{x^2}{2} -2.5x\text{ kN.m} \quad \text{for } 0\le x \le 5\end{split}\]](https://engcourses-uofa.ca/wp-content/ql-cache/quicklatex.com-0f56a2a148160f59271e79b42084c078_l3.png)
7- Determine the functions ![]() and
and ![]() .
.
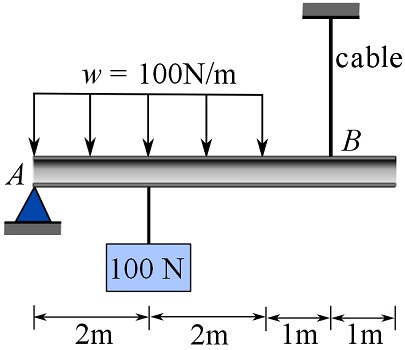
Answer
![Rendered by QuickLaTeX.com \[V(x)=\begin{cases}300 - 100x\text{ N} \quad \text{for } 0< x < 2\\200 -100x \text{ N} \quad \text{for } 2< x \le 4\\-200 \text{ N} \quad \text{for } 4\le x < 5\\0.0\text{ N} \quad \text{for } 5< x \le 6\end{cases}\]](https://engcourses-uofa.ca/wp-content/ql-cache/quicklatex.com-b9e9d9710daceb27b90d32f0abd9f2af_l3.png)
![Rendered by QuickLaTeX.com \[M(x)=\begin{cases}300x - 50x^2\text{ N.m} \quad \text{for } 0\le x \le 2\\200 + 200x - 50x^2\text{ N.m} \quad \text{for } 2\le x \le 4\\-200x + 1000\text{ N.m} \quad \text{for } 4\le x \le 5\\0.0\text{ N.m} \quad \text{for } 5\le x \le 6\end{cases}\]](https://engcourses-uofa.ca/wp-content/ql-cache/quicklatex.com-f8e720e7f033f016061a64c4d1f74a2e_l3.png)
8- For the loaded beam shown, find the locations where the shear force and the bending moment attain their maximum magnitudes. Then, determine the maximum magnitudes. Hint: find the local and global extrema (maxima or minima) of the functions ![]() and
and ![]() . Use derivatives for finding local extrema. You can also plot
. Use derivatives for finding local extrema. You can also plot ![]() and
and ![]() for more clarification.
for more clarification.
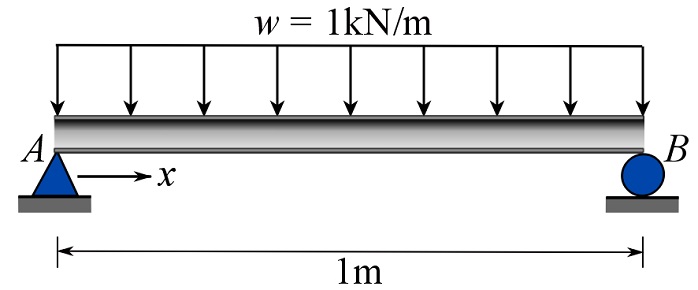
Answer
The maximum magnitude of the shear force is ![]() and it occurs at two locations being the supports, i.e.
and it occurs at two locations being the supports, i.e. ![]() and
and ![]() .
.
The maximum magnitude of the bending moment is ![]() occurring at
occurring at ![]() .
.
9- For the loaded beam shown, find the locations where the shear force and the bending moment attain their maximum magnitudes. Then, determine the maximum magnitudes. Hint: see the previous problem.

Answer
The maximum magnitude of the shear force is ![]() occurring at
occurring at ![]() , i.e. support
, i.e. support ![]() .
.
The maximum magnitude of the bending moment is ![]() occurring at
occurring at ![]() .
.
10- For the loaded beam shown, find the locations where the shear force and the bending moment attain their maximum magnitudes. Then, determine the maximum magnitudes. Hint: see the previous problem.
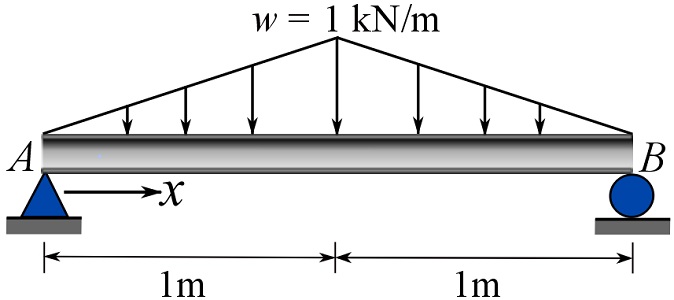
Answer
The maximum magnitude of the shear force is ![]() occurring at
occurring at ![]() and
and ![]() i.e. the supports.
i.e. the supports.
The maximum magnitude of the bending moment is ![]() occurring at
occurring at ![]() .
.
11- For the loaded beam shown, find the locations where the shear force and the bending moment attain their maximum magnitudes. Then, determine the maximum magnitudes. Hint: see the previous problem.
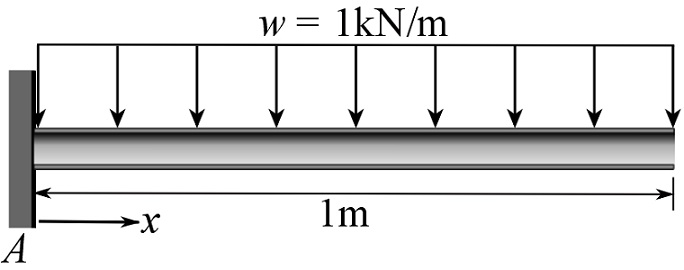
Answer
The maximum magnitude of the shear force is ![]() occurring at
occurring at ![]() i.e. the support.
i.e. the support.
The maximum magnitude of the bending moment is ![]() occurring at
occurring at ![]() i.e. the support.
i.e. the support.
