Engineering at Alberta Courses » Relationships between Load, Shear, and Moments

Internal Forces: Relationships between Load, Shear, and Moments

Direct derivation of the functions $V(x)$ and $M(x)$ by the method of sections may be tedious. The direct application of the method of sections can be avoided by deriving the relationship between the load, shear, and bending moment. In this section, four relationships are derived:
1- point force AND shear
2- distributed load AND shear
3- shear AND bending moment
4- couple moment AND bending moment

1- Point Force and Shear

Consider a downward point force acting at a point $C$ of a beam (Fig. 7.16a). To find the relationship between the shear and the point force, a segment of the beam as shown in Fig. 7.16a is considered. The segment contains the force (point of application) and has a fractional length of $\Delta x$ .

Fig 7.16 Downward point force on a beam.

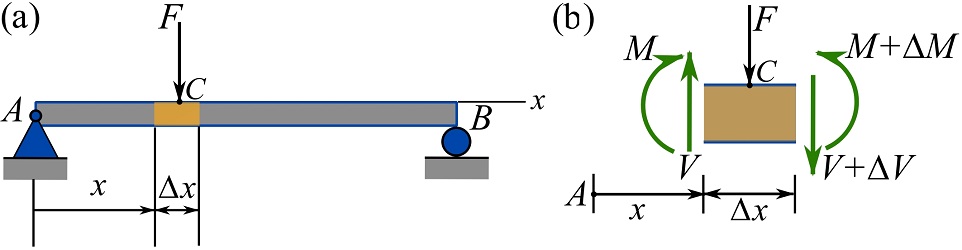
Isolating the segment exposes the internal forces on its FBD as shown in Fig. 7.16b. The beam is free of axial load. The values of the function $V(x)$ and $M(x)$ , evaluated at $x$ , are denoted as $V$ and $M$ respectively. At a distance $x+\Delta x$ away from point $x$ , the shear force and the bending moment generally vary to $V+\Delta V$ and $M+\Delta M$ . The force equilibrium for the free segment is,

$+\uparrow \sum F_y = 0\implies V-F-(V+\Delta V)=0\implies \Delta V=-F$

which can be written as,

(7.1) $\Delta V = -F\quad \text{or}\quad V_{C^+}-V_{C^-}=-F$

where $V_{C^+}$ and $V_{C^-}$ denote the shear right before and after point $C$ respectively.

Equation 7.1 states that a point force $\bold F$ acting on a beam creates a jump, with a magnitude of $F$ , in the values of $V(x)$ . The jump appears as a jump discontinuity in the diagram of $V(x)$ .

Remark: exactly at the application point of a point force, the value of shear is undefined due to the jump discontinuity.

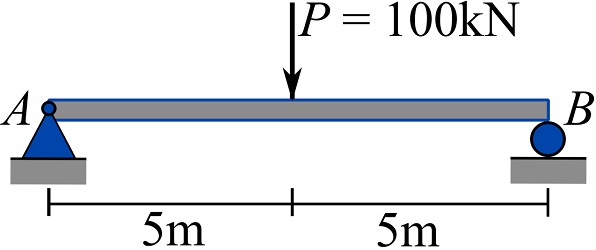
EXAMPLE 7.3.1

Plot the shear diagram of the beam shown. Use Eq. 7.1.

SOLUTION

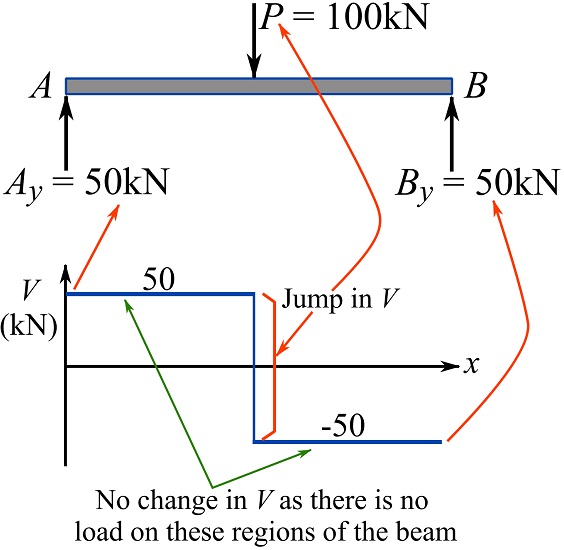
Draw the FBD of the beam and solve for the support reactions.

The FBD with solved support reactions are demonstrated in the figure below.

Start from the known shear at point $A$ and use $\Delta V=-F$ to determine the shear along the beam axis. Observe the jump in the values of $V$ at the point where the external load $P$ acts; the jump in values is $\Delta V=-100$ .

2- Distributed load and Shear

Consider a beam under a continuous distributed load expressed by a function $w(x)$ . As shown in Fig. 7.17a, positive values of $w(x)$ are associated with downward force on the beam.

Fig. 7.17 A beam under a continuous distributed load.

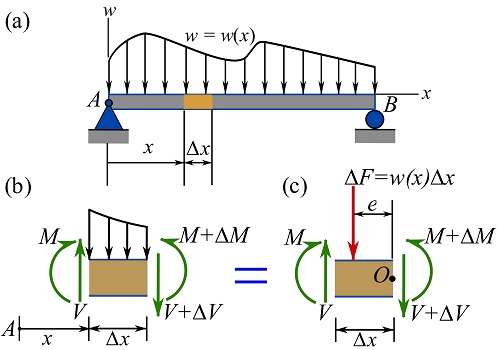
To obtain the relationship between $w(x)$ and $V(x)$ , a beam segment with a fractional length $\Delta x$ is considered at an arbitrary distance $x$ from the left-hand side of the beam (Fig. 7.17a). The FBD of the segment is constructed as shown in Fig 7.17b. The beam is free of axial load. The shear force $V(x)$ and the bending moment $M(x)$ at $x$ are denoted as $V$ and $M$ respectively. The shear force and bending moment at a distance $x+\Delta x$ are denoted as $V+\Delta V$ and $M+\Delta M$ respectively.

As shown in Fig 7.17c, the part of the distributed load, $w(x)$ , acting on the segment is replaced with its (equivalent) resultant force with the magnitude of $\Delta F=w(x)\Delta x$ . The location at which $\Delta F$ acts on the segment is shown by $e$ in the figure. In fact, $\Delta F$ acts somewhere along the length of the segment; in other words, $e=k\Delta x$ where $0<k<1$ . It will be seen that the location of $\Delta F$ does not affect the calculations. Writing the force equation of equilibrium for the FBD of the segment leads to the following relationship.

$+\uparrow \sum F_y = 0\implies V-\Delta F-(V+\Delta V)=0\implies \frac{\Delta V}{\Delta x}=-w(x)$

Therefore, letting $\Delta x \to 0$ and taking the limit of both sides as,

$\lim_{\Delta x \to 0} \frac{\Delta V}{\Delta x}=-w(x)$

leads to,

(7.2) $\frac{dV}{dx}=-w(x)$

This equation indicates that at any point $x$ along the axis of the beam, the slope of the diagram of $V(x)$ equals the negative value of $w(x)$ . This is the geometrical interpretation of Eq. 7.2.

An alternative form of the relationship between $w(x)$ and $V(x)$ is obtained by writing Eq. 7.2 as $dV=-w(x)dx$ and integrating both sides,

$\int_a^bdV=\int_a^b -w(x)dx$

leads to,

(7.3) $V|_{x=b}-V|_{x=a}=\int_a^b -w(x)dx$

where $a$ and $b$ such that $a<b$ are the coordinates of two points $A$ and $B$ on the axis of the beam.

The geometrical interpretation of Eq. 7.3 indicates that the variation of the shear between two points on the beam equals the area (negative value) under the distributed load between the two points.

Equation 7.3 can be utilized to find $V(x)$ . If the shear, $V_A$ is known at a starting point, $x=a$ , then the shear $V(x)$ at any arbitrary point with the coordinate $x>a$ is,

(7.4) $V(x)=V_A - \int_a^x w(t)dt$

Note that the integration variable is a dummy variable and therefore renamed to $t$ .

EXAMPLE 7.3.2

For the loaded beam shown.
1- Plot the shear diagram of the beam. Use Eq. 7.4.
2- Using the geometrical interpretation of the relationship between external load and shear force, show that $V=25\rm kN$ and $V=0\rm kN$ at $x=2.5\rm m$ and $x=5\rm m$ respectively.

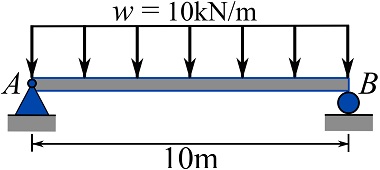
SOLUTION

Part 1

Draw the FBD of the beam and solve for the support reactions.

The FBD with solved support reactions are demonstrated in the figure below.

Starting from point $A$ , determine the shear at any point $x$ using Eq. 7.4 as,

$V(x)=V_A - \int_a^x w(t)dt=50-\int_0^x 10dt=50 -10x\text{ kN}$

Observe the relationship between $w(x)$ and the slope of $V(x)$ ; the slope, $dV/dx$ is constant as $w$ is constant.

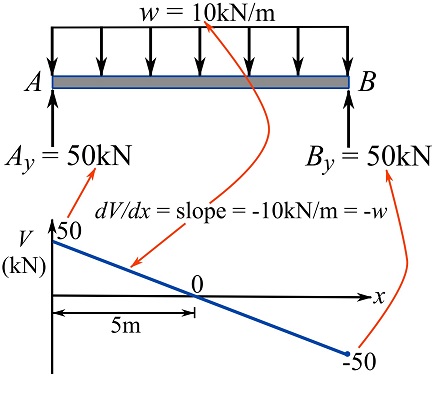
Part 2

Use the relationship $\Delta V=-1(\text {area under } w)$

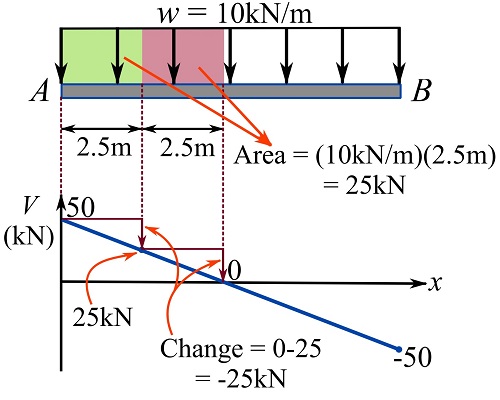
3– Shear and Bending Moment.

Shear and moment relationship can be obtained by writing the moment equation of equilibrium about point $O$ of the free segment shown in Fig. 7.17c as,

$\begin{split}\circlearrowleft{+} \sum M_O=0 &\implies -M-(V)(\Delta x) + (\Delta F)(e)+(M+\Delta M)=0\\&\implies -(V)(\Delta x)+(w(x)\Delta x)(k\Delta x)+\Delta M=0\\&\implies -(V)(\Delta x)+(kw(x))(\Delta x)^2+\Delta M=0\\&\implies V=(kw(x))(\Delta x)+\frac{\Delta M}{\Delta x}\end{split}$

and letting $\Delta x \to 0$ ,

(7.5) $V=\frac{dM}{dx}$

This relationship indicates that at any point $x$ along the axis of the beam, the slope of the diagram of $M(x)$ equals the value of $V(x)$ .

The integral form of Eq. 7.5 is achieved by writing $dM=V(x)dx$ and integrating both sides as,

(7.6) $M|_{x=b}-M|_{x=a}=\int_a^b V(x)dx$

Equation 7.6 indicates that the variation of the bending moment between two points on the beam equals the area under the shear diagram between the two points.

Using Eq. 7.6, the function $M(x)$ can be determined. If the bending moment, $M_A$ is known at a starting point, $x=a$ , then the bending moment $M(x)$ at any arbitrary point with the coordinate $x>a$ is,

(7.7) $M(x)=M_A + \int_a^x V(t)dt$

EXAMPLE 7.3.3

For the loaded beam shown.
1- Plot the moment diagram of the beam. Use Eq. 7.7.
2- Using the geometrical interpretation of the relationship between shear and bending moment, show that $M=250\rm kN.m$ at $x=5\rm m$ .

SOLUTION

Part 1

Drawing the FBD, obtaining the support reactions, and drawing the shear diagram are already done and demonstrated in Example 7.3.1.

To determine $M(x)$ , use Eq. 7.7 for $0\le x\le 5$ and $5\le x\le 10$ .

For $0\le x\le 5$ ,

$\begin{split}M(x)&=M_A + \int_0^x V(t)dt=0+\int_0^x 50dt\\&\therefore M(x)=50x\text{ kN.m}\quad 0\le x\le 5\end{split}$

For $5\le x\le 10$ ,

$\begin{split}M(x)&=M_A + \int_0^x V(t)dt=0+\int_0^5 50dt+\int_5^x -50dt\\&\therefore M(x)=500 -50x \text{ kN.m}\quad 5\le x\le 10\end{split}$

Therefore,

$M(x)=\begin{cases}50x\text{ kN.m}\quad 0\le x\le 5\\-50x+500 \text{ kN.m}\quad 5\le x\le 10\end{cases}$

The diagram of $M(x)$ is as follows,

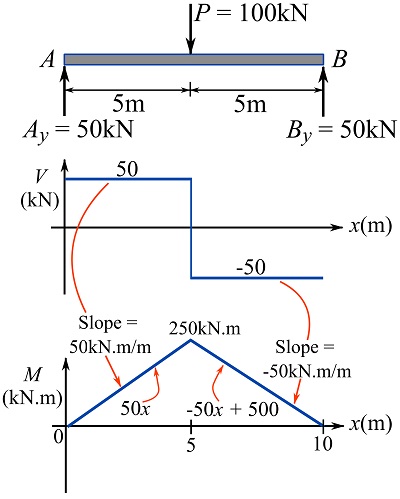
Part 2

Use the relationship $\Delta M=\text {area under } V$ .

$\begin{split}\Delta M &= M(5)-M(0)=(50\text{kN.m})(5\text{m})=250\text{ kN.m}\\&\therefore M(5)= 250\text{ kN.m}\end{split}$

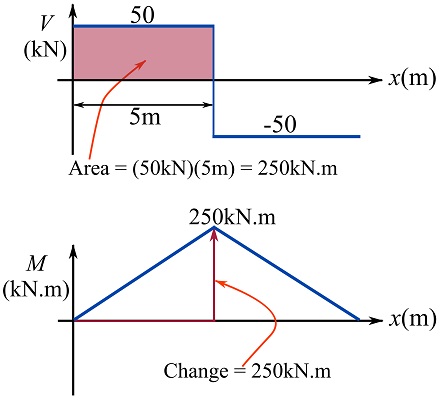
EXAMPLE 7.3.4

SOLUTION

Part 1

Drawing the FBD, obtaining the support reactions, and drawing the shear diagram are already done and demonstrated in Example 7.3.2.

To determine $M(x)$ , use Eq. 7.7 for $0\le x\le 10$ .

$\begin{split}M(x)&=M_A + \int_0^x V(t)dt=0+\int_0^x -10x+50dt\\&\therefore M(x)=-5x^2+50x\text{ kN.m}\end{split}$

The diagram of $M(x)$ is as follows,

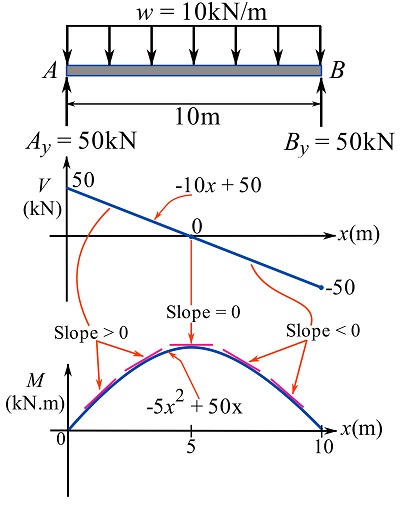
Part 2

Use the relationship $\Delta M=\text {area under } V$ .

$\begin{split}\Delta M&=M(5)-M(0)=(50\text{kN})(5m)/2=125\text{ kN.m}\\&\therefore M(5)= 125\text{ kN.m}\end{split}$

See figure below for the demonstration.

Part 3

$\begin{split}\Delta M&=M(10)-M(5)=(50\text{kN})(5m)/2=125\text{ kN.m}\&\implies 0 - 125= -125\text{ kN.m}\\&\therefore -125=-125\end{split}$

See the above figure for the demonstration.

4- Couple Moment and Bending Moment

Consider an external couple moment acting at a point $O$ of a beam (Fig. 7.18a). To find the relationship between the bending moment and the couple moment, a segment of the beam as shown in Fig. 7.18a is considered.

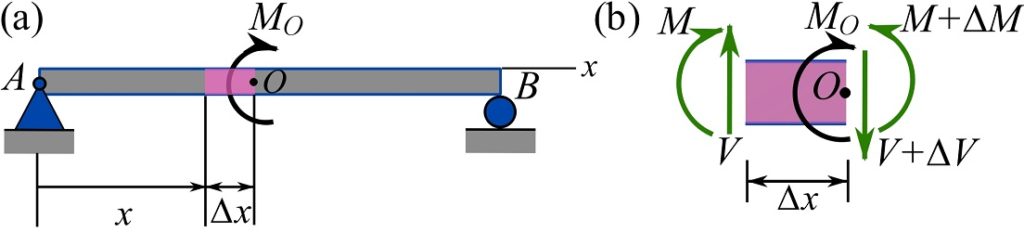
Fig. 7.18 An external couple moment acting on a beam.

The FBD of the segment is shown in Fig 7.18b. Writing the moment equation of equilibrium for the free segments lead to,

$\circlearrowleft{+} \sum (M)_O=0 \implies -M-M_O-(V)(\Delta x) +(M+\Delta M)=0\implies -M_O-(V)(\Delta x) + \Delta M=0$

Letting $\Delta x\to 0$ , results in,

(7.8) $\Delta M = M_O\quad \text{or}\quad M_{O^+}-M_{O^-}=M_O$

where $M_{O^+}$ and $M_{O^-}$ denote the bending moment right before and after point $O$ respectively.

Equation 7.9 states that a couple moment $\bold M$ acting on a beam creates a jump, with a magnitude of $M$ , in the values of $M(x)$ . The jump appears as a jump discontinuity in the diagram of $M(x)$ .

Remark: the value of bending moment exactly at the application point of a couple moment is undefined due to the jump discontinuity.

Use the following interactive tool to obtain SFD and BMD of different beams (simply supported and cantilever beams) with different supports and loads. Observe the variations within each diagram upon changing the loads and other inputs.

Note that the numerical results may contain negligible error due to round off. Also, In some cases you may observe that the support reaction of the roller becomes a pulling force on the beam. In this case, assume that the support is a roller in a frictionless slot

Open Educational Resources

Internal Forces: Relationships between Load, Shear, and Moments

1- Point Force and Shear

EXAMPLE 7.3.1

SOLUTION

2- Distributed load and Shear

EXAMPLE 7.3.2

SOLUTION

3– Shear and Bending Moment.

EXAMPLE 7.3.3

SOLUTION

EXAMPLE 7.3.4

SOLUTION

4- Couple Moment and Bending Moment

Video

Leave a Reply Cancel reply