Engineering at Alberta Courses

Forces and Moments: Couple moment

Moment of a couple

Imagine that you want to turn a valve, or the steering wheel of a car (Fig. 3.20a). You can do it by two hands as one hand pushes up and the other one pulls down the valve or the steering wheel. As a result, the valve or the steering wheel rotates. Your hands (ideally) generated two equal and parallel forces but with opposite directions. This pair of forces creates a turning effect,referred to as a couple formally defined as below.

A couple. A couple is defined as a pair of parallel but noncollinear forces that have the same magnitude but opposite directions (Fig. 3.20b). Each force is referred to as a couple force. Because the lines of action of the couple forces are parallel and noncollinear, they are in the same plane. The (perpendicular) distance, $d$ , between the lines of action of the parallel forces is called the perpendicular distance or moment arm between the forces.

The moment created by a couple is called a couple moment. To find the couple moment of a couple, an arbitrary point, $O$ , in the space is considered and the moment of each couple force is calculated about that point (Fig. 3.21),

$\bold M_O= \bold r_B\times \bold F+ \bold r_A\times (-\bold F)=(\bold r_B-\bold r_A)\times \bold F$

where $\bold r_A$ and $\bold r_B$ are position vectors directed from $O$ to any points (A and B in this case) on the lines of action of the forces. Let $\bold r = \bold r_B-\bold r_A$ . Then, $r$ is a position vector linking the two points on the lines of actions. According to the choices of $A$ and $B$ , $\bold r$ is obviously independent of the choice of $O$ ; therefore, the couple moment becomes independent of $O$ and also the position vectors $\bold r_A$ and $\bold r_B$ . Thereby, the couple moment is formulated as,

(3.20) $\bold M=\bold r \times \bold F$

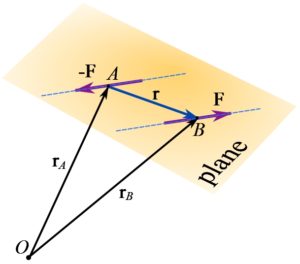
Equation 3.20 indicates that a couple moment of a particular couple only depends on the forces and their distance and does not depend on the point about which the couple moment is calculated. Therefore, a couple moment (vector) is not assigned to any point and can be freely positioned anywhere in the space. Such a vector is called a free vector.

Remark: note the difference between the terms the moment of a force about a point and the moment of a couple.

Remark: a force is a sliding vector meaning that it can slide along its line of action without affecting the calculations. A couple moment is a free vector meaning that it can be placed anywhere in the space or the body without affecting the calculations.

A couple moment can be determined using both scalar and vector formulations:

Scalar formulation. Since the forces of a couple are coplanar (Fig 3.22), the magnitude of couple moment can be determined by summing the moment of each force about an arbitrary point, $O$ , in the plane as demonstrated in Fig. 3.22,

(3.21) $M=(F)(d_1) +(F)(d_2)=F(d_1+d_2)=Fd$

where $d_1$ and $d_2$ are the moments arms (perpendicular distance) of the forces.

Determined by the right-hand rule, the direction of the couple moment is perpendicular to the plane of the couple forces and the sense of rotation follows the fingers curled (Fig. 3.22).

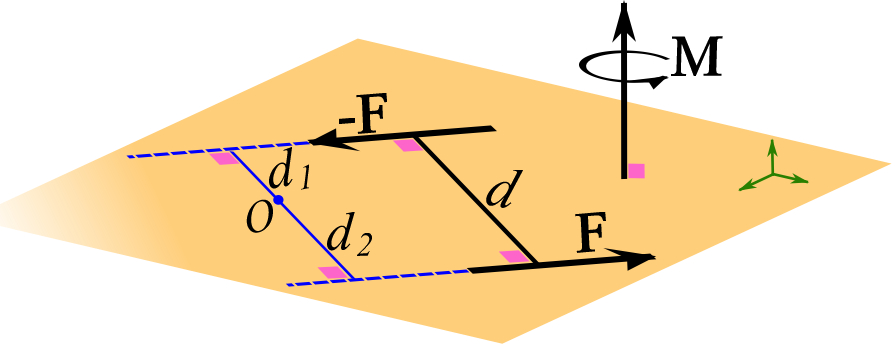
Fig. 3.22 A couple moment and its direction.

Calculation of Eq. 3.21 can be interpreted as the moment of one of the forces about a point on the line of action of the other force.

Vector formulation. The vector formulation is in fact Eq. 3.20. This formulation is used when a couple moment is needed to be written in its vector form. Like the scalar formulation, the vector formulation can be interpreted as calculating the moment of one of the couple forces about a point on the line of action of the other force.