Mathematical Preliminaries
Mathematical Symbols:
The following are the mathematical symbols used throughout these online material.
- Curly brackets are used to designate sets. For example
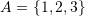 is the set of the three elements 1, 2, and 3.
is the set of the three elements 1, 2, and 3. - We use the symbol
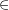 to designate elements in sets. For example, if
to designate elements in sets. For example, if 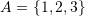 , then
, then 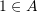 .
. - The symbol
 means “subset of” and is used to indicate that the elements of one set are elements of another set. For example, if
means “subset of” and is used to indicate that the elements of one set are elements of another set. For example, if 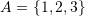 and
and 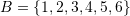 , then
, then 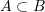 .
. - Sets can be defined using properties listed within the curly brackets. For example, we can define the set
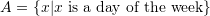 . This means that
. This means that 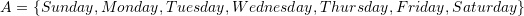 .
. 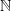 is used to designate the set of natural numbers starting from 1. i.e.,
is used to designate the set of natural numbers starting from 1. i.e., 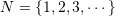 .
. is used to designate the set of integers. i.e.,
is used to designate the set of integers. i.e., 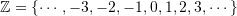 .
.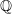 is used to designate the set of rational numbers. i.e.,
is used to designate the set of rational numbers. i.e., 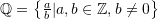 .
.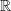 is used to designate the set of real numbers.
is used to designate the set of real numbers.- Box brackets are used to designate (closed) intervals in real numbers. For example,
![Rendered by QuickLaTeX.com A=[0,1]](https://engcourses-uofa.ca/wp-content/ql-cache/quicklatex.com-838b321ec17052577240af06f4f1bb76_l3.png) is the set of real numbers between 0 and 1 and including both 0 and 1.
is the set of real numbers between 0 and 1 and including both 0 and 1. - Round brackets are used to designate (open) intervals in real numbers. for example,
![Rendered by QuickLaTeX.com B=(0,1]](https://engcourses-uofa.ca/wp-content/ql-cache/quicklatex.com-1b1e12408ab515c5d0004819c9fcb9f0_l3.png) is the set of real numbers between 0 and 1 and including 1.
is the set of real numbers between 0 and 1 and including 1. - The symbol
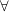 means “for every” and is usually used to designate a property of all the elements of a set. For example, if we say that
means “for every” and is usually used to designate a property of all the elements of a set. For example, if we say that 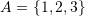 , we can describe the fact that all the elements in
, we can describe the fact that all the elements in 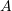 are greater than zero by stating:
are greater than zero by stating: 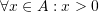 .
. - The symbol
 means “exists” and is used to identify particular elements in sets. For example, if
means “exists” and is used to identify particular elements in sets. For example, if 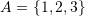 we can say that
we can say that 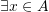 such that
such that  , i.e., there exists an element in the set
, i.e., there exists an element in the set 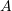 which is greater than 0.
which is greater than 0. - The symbol
 means “exists and unique” and is used to identify a unique element in a set. For example, if
means “exists and unique” and is used to identify a unique element in a set. For example, if 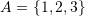 we can say that
we can say that 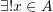 such that
such that 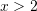 , i.e., there exists one element and one element only in the set
, i.e., there exists one element and one element only in the set 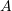 which is greater than 2.
which is greater than 2. - To define functions or maps between sets, we use
 . If
. If 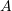 is a set and
is a set and 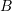 is another set, then a function that maps elements of
is another set, then a function that maps elements of 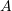 into elements of
into elements of 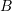 is defined with the notation
is defined with the notation 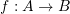 .
. - If
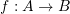 , then, the arrow
, then, the arrow  is used to designate that a function
is used to designate that a function 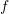 maps the element
maps the element 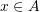 to
to 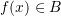 as follows
as follows 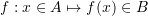 .
. - The symbol
 is used in many contexts. One of them is the cross product which will be defined later. Another is for defining the set of ordered pairs. For example, if
is used in many contexts. One of them is the cross product which will be defined later. Another is for defining the set of ordered pairs. For example, if 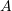 and
and 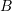 are two sets. Then, the set
are two sets. Then, the set 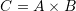 is the set made of ordered pairs
is the set made of ordered pairs 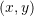 with
with 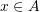 and
and 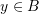 . For example if
. For example if 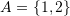 and
and 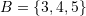 , then
, then 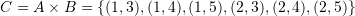 . The set
. The set 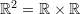 is the set of ordered pairs of real numbers and geometrically designates a plane. Similarly,
is the set of ordered pairs of real numbers and geometrically designates a plane. Similarly,  is the set representing the three dimensional space.
is the set representing the three dimensional space. 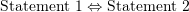 is used to designate equivalence of statements 1 and 2(if and only if). For example:
is used to designate equivalence of statements 1 and 2(if and only if). For example: 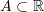 is equivalent to
is equivalent to 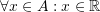 . So, this can be written as:
. So, this can be written as: 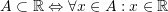 .
.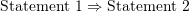 is used to describe that statements 1 leads to statement 2. For example:
is used to describe that statements 1 leads to statement 2. For example: 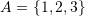 leads to the statement
leads to the statement 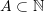 . So, this can be written as:
. So, this can be written as: 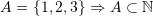 .
.- The Kronecker delta
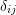 is a special symbol which gives a value of either 0 or 1 depending on the subscripts
is a special symbol which gives a value of either 0 or 1 depending on the subscripts  and
and 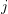 . Usually
. Usually  and
and 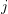 can take values of 1, 2, or 3 and
can take values of 1, 2, or 3 and
![Rendered by QuickLaTeX.com \[ \delta_{ij}= \begin{cases} 1 & i=j\\ 0 & i\neq j \end{cases} \]](https://engcourses-uofa.ca/wp-content/ql-cache/quicklatex.com-7a359205b3390e5276f42ab3fdfc0887_l3.png)
- The alternator
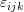 is a special symbol which gives a value of either 0, 1, or -1 depending on the subscripts
is a special symbol which gives a value of either 0, 1, or -1 depending on the subscripts  ,
, 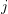 , and
, and 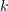 . Usually
. Usually  ,
, 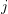 , and
, and 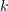 can take values of 1, 2, or 3 and
can take values of 1, 2, or 3 and
![Rendered by QuickLaTeX.com \[ \varepsilon_{ijk}= \begin{cases} 1 & i,j,k \mbox{ is a cyclic permutation of 1, 2, 3}\\ -1 & i,j,k \mbox{ is a noncyclic permutation of 1, 2, 3}\\ 0 & \mbox{Otherwise} \end{cases} \]](https://engcourses-uofa.ca/wp-content/ql-cache/quicklatex.com-f28ef771f996180bf4032b4cdefa7b2c_l3.png)
- Unless stated explicitly, we do not differentiate between the column or row representation of vectors in
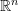 . The curly brackets are also used for the row representation of vectors to be consistent with how Mathematica defines a vector. Therefore, the following are equivalent for the three dimensional vector
. The curly brackets are also used for the row representation of vectors to be consistent with how Mathematica defines a vector. Therefore, the following are equivalent for the three dimensional vector  with components
with components 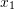 ,
, 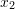 , and
, and 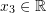 :
:
![Rendered by QuickLaTeX.com \[ x=\left(\begin{array}{c}x_1\\x_2\\x_3\end{array}\right)=\{x_1,x_2,x_3\}=(x_1,x_2,x_3) \]](https://engcourses-uofa.ca/wp-content/ql-cache/quicklatex.com-89d6adac461112e2847a6eea383217eb_l3.png)
The Set of Real Numbers:
The properties of the set of real numbers ![]() is crucial for the study of continuum mechanics. In essence, the set of real numbers
is crucial for the study of continuum mechanics. In essence, the set of real numbers ![]() is the link between what we call a “number” and the quantity along a continuum. An interested reader should consult with the Wikipedia page on real numbers. The development of the real numbers is a prerequisite for the mathematical description of a continuum. An intuitive illustration for this is to think of the continuous line geometrically as a continuum such that between any two points on the line, there is always another point. Similarly, the real numbers is this representation since for every two real numbers, there is always another real number lying in between. This analogy allows the representation of the line using
is the link between what we call a “number” and the quantity along a continuum. An interested reader should consult with the Wikipedia page on real numbers. The development of the real numbers is a prerequisite for the mathematical description of a continuum. An intuitive illustration for this is to think of the continuous line geometrically as a continuum such that between any two points on the line, there is always another point. Similarly, the real numbers is this representation since for every two real numbers, there is always another real number lying in between. This analogy allows the representation of the line using ![]() . The following properties of
. The following properties of ![]() are going to be used and extended to linear vector spaces in linear algebra:
are going to be used and extended to linear vector spaces in linear algebra:
The Set of Real Numbers is Closed under Addition:
If two real numbers are added, the result is always another real number. So, we say that the set of real numbers is closed under addition.
The Set of Real Numbers is Closed under Multiplication:
If two real numbers are multiplied, the result is always another real number. So, we say that the set of real numbers is closed under multiplication.
The Set of Real Numbers Has a “Zero” Element:
0 is a unique element of the real numbers. It is the neutral element of addition since if any element is added to zero, the result is that element. i.e., ![]() .
.
Every Element in the Real Numbers Has an Inverse Element of Addition:
Indeed, if ![]() , then
, then ![]() and
and ![]() .
.
Types of Maps:
Maps or functions between sets can be injective, surjective, bijective, or none of the above.
Injective Maps:
An injective map also called “one-to-one” is a map that designates a unique image for every element. Let ![]() .
. ![]() is injective if
is injective if ![]() such that
such that ![]() .
.
Surjective Maps:
A surjective map also called “onto” is a map such that every element in the codomain has a pre-image. Let ![]() .
. ![]() is surjective if
is surjective if ![]() such that
such that ![]() . In other words, there is always a preimage for all the elements in
. In other words, there is always a preimage for all the elements in ![]() .
.
Bijective Maps:
A bijective map also called “invertible” is a map such which is both injective and surjective. An bijective maps is a map that can be inverted, i.e., let ![]() .
. ![]() is bijective, then, there exists
is bijective, then, there exists ![]() such that
such that ![]() . I.e.,
. I.e., ![]() .
.
Examples:
For example, let ![]() and
and ![]() . The map
. The map ![]() assigns every student a unique ID in the set of IDs of students at the University of Alberta. This map is injective or one-to-one since each student has his/her own unique ID. However, this map is not surjective (onto) because there are IDs in
assigns every student a unique ID in the set of IDs of students at the University of Alberta. This map is injective or one-to-one since each student has his/her own unique ID. However, this map is not surjective (onto) because there are IDs in ![]() that are not assigned to students in
that are not assigned to students in ![]() .
.
Similarly, let ![]() and
and ![]() . There is a surjective map between the students in the class and the gender types as there are both male and female students taking CivE 398. Unless there is only one male student and one female student, this map is surjective but not injective. Figure 1 contains some illustrative examples of the above definitions.
. There is a surjective map between the students in the class and the gender types as there are both male and female students taking CivE 398. Unless there is only one male student and one female student, this map is surjective but not injective. Figure 1 contains some illustrative examples of the above definitions.
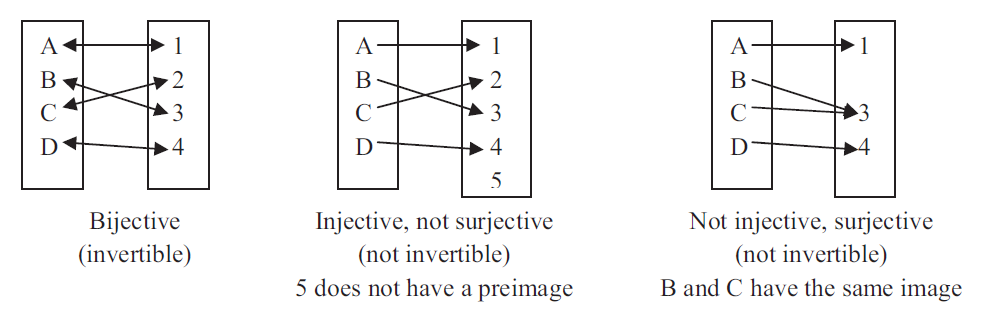
Figure 1. Bijective, injective, and surjective maps
Problems:
- What do the following sets represent?
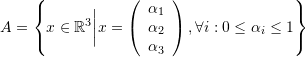 .
.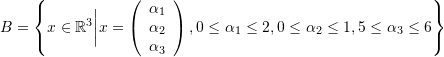 .
.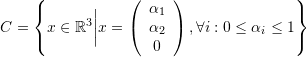 .
.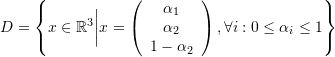 .
.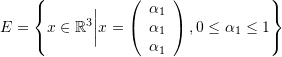 .
.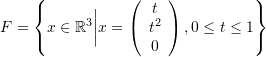 .
.
- What is wrong with the following statements? Can you correct them?
- Statement 1: Samer is from Egypt. Statement 2: Samer is from Africa.
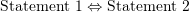 .
. - Statement 1: John is North American. Statement 2: John is Canadian.
 .
.
- Statement 1: Samer is from Egypt. Statement 2: Samer is from Africa.
- If
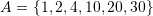 ,
, 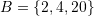 , and
, and 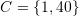 , then which of the following is true and which is false:
, then which of the following is true and which is false:
- Which of the following functions are injective but not surjective, surjective but not injective, bijective, none of the above:
where
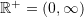
- Find a function
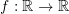 that is injective but not surjective.
that is injective but not surjective. - Find a function
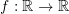 that is surjective but not injective.
that is surjective but not injective.
